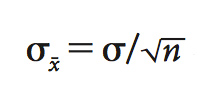A typical lawn sprinkler features various nozzles arranged at angles on a rotating wheel; when water is pumped in, they release jets that cause the wheel to rotate. But what would happen if the water were sucked into the sprinkler instead? In which direction would the wheel turn then, or would it even turn at all? That’s the essence of the “reverse sprinkler” problem that physicists like Richard Feynman, among others, have grappled with since the 1940s. Now, applied mathematicians at New York University think they’ve cracked the conundrum. […]

“We found that the reverse sprinkler spins in the ‘reverse’ or opposite direction when taking in water as it does when ejecting it, and the cause is subtle and surprising.” […] found that the reverse sprinkler rotates a good 50 times slower than a regular sprinkler, but it operates along similar mechanisms, which is surprising. […]

The reverse sprinkler problem is associated with Feynman because he popularized the concept, but it actually dates back to a chapter in Ernst Mach’s 1883 textbook The Science of Mechanics (Die Mechanik in Ihrer Entwicklung Historisch-Kritisch Dargerstellt). Mach’s thought experiment languished in relative obscurity until a group of Princeton University physicists began debating the issue in the 1940s.

{ ArsTechnica | Continue reading }

acrylic on canvas { David Hockney, A Lawn Being Sprinkled, 1967 }

John von Neumann (1903 – 1957) was a Hungarian-American mathematician, physicist, computer scientist, engineer and polymath. Von Neumann was generally regarded as the foremost mathematician of his time. He integrated pure and applied sciences.

Von Neumann made major contributions to many fields, including mathematics (foundations of mathematics, functional analysis, ergodic theory, representation theory, operator algebras, geometry, topology, and numerical analysis), physics (quantum mechanics, hydrodynamics, and quantum statistical mechanics), economics (game theory), computing (Von Neumann architecture, linear programming, self-replicating machines, stochastic computing), and statistics. […]

He first proposed a quantum logic in 1932 […] He founded the field of game theory as a mathematical discipline. […] His analysis of the structure of self-replication preceded the discovery of the structure of DNA. […] He made fundamental contributions to mathematical statistics and in the field of fluid dynamics. […] He was a founding figure in computing. […] Beginning in 1949, von Neumann’s design for a self-reproducing computer program is considered the world’s first computer virus, and he is considered to be the theoretical father of computer virology. […] Von Neumann and his appointed assistant on this project, Jule Gregory Charney, wrote the world’s first climate modelling software, and used it to perform the world’s first numerical weather forecasts. […] The first use of the concept of a singularity in the technological context is attributed to von Neumann. […]

Beginning in the late 1930s, von Neumann developed an expertise in explosions—phenomena that are difficult to model mathematically. During this period, von Neumann was the leading authority of the mathematics of shaped charges. This led to his involvement in the Manhattan Project. […] He made his principal contribution to the atomic bomb in the concept and design of the explosive lenses that were needed to compress the plutonium core of the Fat Man weapon that was later dropped on Nagasaki. […] As a Hungarian émigré, concerned that the Soviets would achieve nuclear superiority, he designed and promoted the policy of mutually assured destruction to limit the arms race. […]

Von Neumann was a child prodigy. When he was six years old, he could divide two eight-digit numbers in his head and could converse in Ancient Greek. When the six-year-old von Neumann caught his mother staring aimlessly, he asked her, “What are you calculating?” […]

Nobel Laureate Hans Bethe said “I have sometimes wondered whether a brain like von Neumann’s does not indicate a species superior to that of man”, and later Bethe wrote that “[von Neumann’s] brain indicated a new species, an evolution beyond man”. [… Israel Halperin said: “Keeping up with him was … impossible. The feeling was you were on a tricycle chasing a racing car.” […]

Von Neumann was also noted for his eidetic memory (sometimes called photographic memory). […] “He was able on once reading a book or article to quote it back verbatim; moreover, he could do it years later without hesitation. He could also translate it at no diminution in speed from its original language into English.”

{ Wikipedia | Continue reading }

oil on canvas { Gustave Caillebotte, Un balcon, boulevard Haussmann, 1888 }

Use each of the numbers 1, 3, 4, and 6 exactly once with any of the four basic math operations (addition, subtraction, multiplication, and division) to total 24. Each number must be used once and only once, and you may define the order of operations; for example, 3 * (4 + 6) + 1 = 31 is valid, however incorrect, since it doesn’t total 24.

{ solution | Hacking, The art of Exploitation | PDF }

The Principia Mathematica (PM) is a three-volume work on the foundations of mathematics written by Alfred North Whitehead and Bertrand Russell and published in 1910, 1912, and 1913.

was an attempt to describe a set of axioms and inference rules in symbolic logic from which all mathematical truths could in principle be proven.

PM has long been known for its typographical complexity. Famously, several hundred pages of PM precede the proof of the validity of the proposition 1+1=2.

{ Wikipedia | Continue reading }

If you write clearly, then your readers may understand your mathematics and conclude that it isn’t profound. Worse, a referee may find your errors. Here are some tips for avoiding these awful possibilities.

1. Never explain why you need all those weird conditions, or what they mean. For example, simply begin your paper with two pages of notations and conditions without explaining that they mean that the varieties you are considering have zero-dimensional boundary. In fact, never explain what you are doing, or why you are doing it. The best-written paper is one in which the reader will not discover what you have proved until he has read the whole paper, if then

2. Refer to another obscure paper for all the basic (nonstandard) definitions you use, or never explain them at all. This almost guarantees that no one will understand what you are talking about

[…]

11. If all else fails, write in German.

{ J.S. Milne | Continue reading }

photos { Left: William Henry Jackson, Pike’s Peak from the Garden of the Gods, Colorado Midland Series, ca.1880 | Right: Ye Rin Mok }

Two mathematicians have uncovered a simple, previously unnoticed property of prime numbers — those numbers that are divisible only by 1 and themselves. Prime numbers, it seems, have decided preferences about the final digits of the primes that immediately follow them.

Among the first billion prime numbers, for instance, a prime ending in 9 is almost 65 percent more likely to be followed by a prime ending in 1 than another prime ending in 9. In a paper posted online today, Kannan Soundararajan and Robert Lemke Oliver of Stanford University present both numerical and theoretical evidence that prime numbers repel other would-be primes that end in the same digit, and have varied predilections for being followed by primes ending in the other possible final digits. […]

This conspiracy among prime numbers seems, at first glance, to violate a longstanding assumption in number theory: that prime numbers behave much like random numbers. Most mathematicians would have assumed, Granville and Ono agreed, that a prime should have an equal chance of being followed by a prime ending in 1, 3, 7 or 9 (the four possible endings for all prime numbers except 2 and 5).

{ Quanta | Continue reading }

In an update on an old story, an investment banker asks the client to pay by placing one penny on the first square of a chessboard, two pennies on the second square, four on the third, doubling the number on each square that follows. If the banker had asked for this on only the white squares, the initial penny would double thirty-one times to $21,474,836 on the last square. Using both the black and the white squares, the sum on the last square is $92,233,720,368,547,758.

People are reasonably good at estimating how things add up, but for compounding, which involved repeated multiplication, we fail to appreciate how quickly things grow. As a result, we often lose sight of how important even small changes in the average rate of growth can be. For an investment banker, the choice between a payment that doubles with every square on the chessboard and one that doubles with every other square is more important than any other part of the contract. […]

Growth rates for nations drive home the point that modest changes in growth rates are possible and that over time, these have big effects. […]

If economic growth could be achieved only by doing more and more of the same kind of cooking, we would eventually run out of raw materials and suffer from unacceptable levels of pollution and nuisance. Human history teaches us, however, that economic growth springs from better recipes, not just from more cooking.

{ Paul Romer | Continue reading }

Statisticians love to develop multiple ways of testing the same thing. If I want to decide whether two groups of people have significantly different IQs, I can run a t-test or a rank sum test or a bootstrap or a regression. You can argue about which of these is most appropriate, but I basically think that if the effect is really statistically significant and large enough to matter, it should emerge regardless of which test you use, as long as the test is reasonable and your sample isn’t tiny. An effect that appears when you use a parametric test but not a nonparametric test is probably not worth writing home about.

A similar lesson applies, I think, to first dates. When you’re attracted to someone, you overanalyze everything you say, spend extra time trying to look attractive, etc. But if your mutual attraction is really statistically significant and large enough to matter, it should emerge regardless of the exact circumstances of a single evening. If the shirt you wear can fundamentally alter whether someone is attracted to you, you probably shouldn’t be life partners. […]

In statistical terms, a glance at across a bar doesn’t give you a lot of data and increases the probability you’ll make an incorrect decision. As a statistician, I prefer not to work with small datasets, and similarly, I’ve never liked romantic environments that give me very little data about a person. (Don’t get me started on Tinder. The only thing I can think when I see some stranger staring at me out of a phone is, “My errorbars are huge!” which makes it very hard to assess attraction.) […]

I think there’s even an argument for being deliberately unattractive to your date, on the grounds that if they still like you, they must really like you.

{ Obsession with Regression | Continue reading }

Imagine that you are imprisoned in a tunnel that opens out onto a precipice two paces to your left, and a pit of vipers two paces to your right. To torment you, your evil captor forces you to take a series of steps to the left and right. You need to devise a series that will allow you to avoid the hazards — if you take a step to the right, for example, you’ll want your second step to be to the left, to avoid falling off the cliff. You might try alternating right and left steps, but here’s the catch: You have to list your planned steps ahead of time, and your captor might have you take every second step on your list (starting at the second step), or every third step (starting at the third), or some other skip-counting sequence. Is there a list of steps that will keep you alive, no matter what sequence your captor chooses?

In this brainteaser, devised by the mathematics popularizer James Grime, you can plan a list of 11 steps that protects you from death. But if you try to add a 12th step, you are doomed: Your captor will inevitably be able to find some skip-counting sequence that will plunge you over the cliff or into the viper pit.

Around 1932, Erdős asked, in essence, what if the precipice and pit of vipers are three paces away instead of two? What if they are N paces away? Can you escape death for an infinite number of steps? The answer, Erdős conjectured, was no — no matter how far away the precipice and viper pit are, you can’t elude them forever.

But for more than 80 years, mathematicians made no progress on proving Erdős’ discrepancy conjecture (so named because the distance from the center of the tunnel is known as the discrepancy).

{ Quanta | Continue reading }

In a patent dispute between two pharmaceutical giants arguing over who owns the royalty rights to a lucrative wound-dressing solution, […] three judges coined a new legal definition of “one”. […]

The ConvaTec patent covered any salt solution “between 1 per cent and 25 per cent of the total volume of treatment”. However, Smith & Nephew devised a competing product that used 0.77 per cent concentration, bypassing, or so it believed, the ConvaTec patent. […]

Their lordships concluded that “one” includes anything greater or equal to 0.5 and less than 1.5  – much to the chagrin of Smith & Nephew.

{ The Independent | Continue reading }

An interesting idea is that the universe could be spontaneously created from nothing, but no rigorous proof has been given. In this paper, we present such a proof based on the analytic solutions of the Wheeler-DeWitt equation.

{ arXiv | Continue reading | more }

Can you ever be reasonably sure that something is random, in the same sense you can be reasonably sure something is not random (for example, because it consists of endless nines)? Even if a sequence looked random, how could you ever rule out the possibility that it had a hidden deterministic pattern? And what exactly do we mean by “random,” anyway?

These questions might sound metaphysical, but we don’t need to look far to find real-world consequences. In computer security, it’s crucial that the keys used for encryption be generated randomly—or at least, randomly enough that a potential eavesdropper can’t guess them. Day-to-day fluctuations in the stock market might look random—but to whatever extent they can be predicted, the practical implications are obvious. Casinos, lotteries, auditors, and polling firms all get challenged about whether their allegedly random choices are really random, and all might want ways to reassure skeptics that they are.

Then there’s quantum mechanics, which famously has declared for a century that “God plays dice,” that there’s irreducible randomness even in the behavior of subatomic particles.

{ American Scientist | Continue reading }

image { Matt Waples }

A computer has solved the longstanding Erdős discrepancy problem. Trouble is, we have no idea what it’s talking about — because the solution, which is as long as all of Wikipedia’s pages combined, is far too voluminous for us puny humans to confirm.

A few years ago, the mathematician Steven Strogatz predicted that it wouldn’t be too much longer before computer-assisted solutions to math problems will be beyond human comprehension.

{ io9 | Continue reading }

photo { Taryn Simon }

A set of mathematical laws that I call the Improbability Principle tells us that we should not be surprised by coincidences. In fact, we should expect coincidences to happen.

One of the key strands of the principle is the law of truly large numbers. This law says that given enough opportunities, we should expect a specified event to happen, no matter how unlikely it may be at each opportunity. Sometimes, though, when there are really many opportunities, it can look as if there are only relatively few. This misperception leads us to grossly underestimate the probability of an event: we think something is incredibly unlikely, when it’s actually very likely, perhaps almost certain.

{ Scientific American | Continue reading }

George E. P. Box is famous for the quote: “all models are wrong, but some are useful.” […]

In my experience, most models outside of physics are heuristic models. The models are designed as caricatures of reality, and built to be wrong while emphasizing or communicating some interesting point. Nobody intends these models to be better and better approximations of reality, but a toolbox of ideas. Although sometimes people fall for their favorite heuristic models, and start to talk about them as if they are reflecting reality, I think this is usually just a short lived egomania. As such, pointing out that these models are wrong is an obvious statement: nobody intended them to be not wrong. Usually, when somebody actually calls such a model “wrong” they actually mean “it does not properly highlight the point it intended to” or “the point it is highlighting is not of interest to reality”. As such, if somebody says that your heuristic model is wrong, they usually mean that it’s not useful and Box’s defense is of no help.

On the opposite end of the spectrum are abstractions, these sort of models are rigorous mathematical statements about specific types of structures. These models are right and true of their subjects in any reasonable definition of the words. They are as right or true as the statement that there are infinite number of primes; or that in Euclidean geometry, the tree angles of a triangle sum to two right angles. When somebody says that an abstraction is wrong, they mean one of two things:

1. It is mathematically false. […]

2. Or, the structure you are applying it to does not meet the requirements of the abstraction. For example, in general relativity, space is non-Euclidean, so triangles don’t sum to 180 degrees.

{ Theory, Evolution, and Game Groups | Continue reading }

I think you are missing out on some ideas on complexity. […] What makes you think that something mathematical is comprehensible? You already invoked one simple form of incomprehension: undecidability in computing. […] As to a belief that the universe is not “mathematical”: well, what else could it possibly be? Many mathematicians define mathematics as the sum-total of all possibility; to say that something isn’t mathematical is tantamount to saying it isn’t possible. Since there is nothing else that it could be, by law of excluded middle, it must be.

{ Linas Vepstas | Continue reading }

Hmmm, no edit-button to correct my post. Some footnotes, then: […]

Box’s quote is kind-of the mirror image of Kolmogorov complexity, which states that a model is useful only if it is smaller than the thing being modelled, and, what’s more, that there are things that cannot be modeled.

{ Linas Vepstas | Continue reading }

Another power law […] is Benford’s Law, which states that the distribution of digits in a lot of data are not even, but power law distributed. For example, in base 10, the number one should, all things being equal, appear 10% of the time. But in many data sources one appears around 30% of the time. This fact is actually used to help detect fraud in, for example, tax returns.

{ Oscillatory Thoughts | Continue reading | NY Times }

On August 31, 2012, Japanese mathematician Shinichi Mochizuki posted four papers on the Internet.

The titles were inscrutable. The volume was daunting: 512 pages in total. The claim was audacious: he said he had proved the ABC Conjecture, a famed, beguilingly simple number theory problem that had stumped mathematicians for decades. […]

The problem, as many mathematicians were discovering when they flocked to Mochizuki’s website, was that the proof was impossible to read. The first paper, entitled “Inter-universal Teichmuller Theory I: Construction of Hodge Theaters,” starts out by stating that the goal is “to establish an arithmetic version of Teichmuller theory for number fields equipped with an elliptic curve…by applying the theory of semi-graphs of anabelioids, Frobenioids, the etale theta function, and log-shells.”

This is not just gibberish to the average layman. It was gibberish to the math community as well.

{ Caroline Chen/Project Wordsworth | Continue reading }

art { Cy Twombly, Coronation of Sesostris, 2000 }

“What people do in cities—create wealth, or murder each other—shows a relationship to the size of the city, one that isn’t tied just to one era or nation,” says Lobo. The relationship is captured by an equation in which a given parameter—employment, say—varies exponentially with population. In some cases, the exponent is 1, meaning whatever is being measured increases linearly, at the same rate as population. Household water or electrical use, for example, shows this pattern; as a city grows bigger its residents don’t use their appliances more. […]

If the population of a city doubles over time, or comparing one big city with two cities each half the size, gross domestic product more than doubles. Each individual becomes, on average, 15 percent more productive. Bettencourt describes the effect as “slightly magical,” although he and his colleagues are beginning to understand the synergies that make it possible. Physical proximity promotes collaboration and innovation, which is one reason the new CEO of Yahoo recently reversed the company’s policy of letting almost anyone work from home. […]

Remarkably, this phenomenon applies to cities all over the world, of different sizes, regardless of their particular history, culture or geography. Mumbai is different from Shanghai is different from Houston, obviously, but in relation to their own pasts, and to other cities in India, China or the U.S., they follow these laws.

{ Smithsonian | Continue reading }

art { Alex Roulette }

Find the pattern for the following series of numbers:

8, 5, 4, 9, 1, 7, 6, 3, 2, 0

{ Solution }

What constitutes a dangerous equation? […] Few would disagree that the obvious winner in this contest would be Einstein’s iconic equation

E=MC²

for it provides a measure of the enormous energy hidden within ordi- nary matter. Its destructive capability was recognized by Leo Szilard, who then instigated the sequence of events that culminated in the construction of atomic bombs.

This is not, however, the direction I wish to pursue. Instead, I am interested in equations that unleash their danger, not when we know about them, but rather when we do not; equations that allow us to understand things clearly, but whose absence leaves us dangerously ignorant.* There are many plausible candidates that seem to fill the bill. But I feel that there is one that surpasses all others in the havoc wrought by ignorance of it over many centuries. It is the equation that provides us with the standard deviation of the sampling distribution of the mean; what might be called De Moivre’s equation:

For those unfamiliar with De Moivre’s equation let me offer a brief aside to explain it.

Why have I decided to choose this simple equation as the most dangerous? There are three reasons, related to

(1) the extreme length of time during which ignorance of it has caused confusion,
(2) the wide breadth of areas that have been misled, and
(3) the seriousness of the consequences that such ignorance has
caused.

{ Howard Wainer | PDF }

photo { Adam Broomberg & Oliver Chanarin }