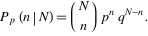During World War II, Allied forces readily admitted German tanks were superior to their own. The big question for Allied forces, then, was how many tanks Germany was producing. Knowing that would help them counter the threat. Here’s how they reverse-engineered serial numbers to find out.

To solve the problem of determining production numbers, Allied forces initially tried conventional intelligence gathering: spying, intercepting and decoding transmissions and interrogating captured enemies.

Using these methods, the Allies deduced that the German military industrial complex churned out around 1,400 tanks each month from June 1940 through September 1942. That just didn’t seem right.

To put that number in context, Axis forces used 1,200 tanks during the Battle of Stalingrad, an eight month battle that resulted in almost two million casualties. That meant the estimate of 1,400 most likely was too high.

Obviously skeptical of that result, the Allies looked for other methods of estimation. That’s when they found a critical clue: serial numbers.

Allied intelligence noticed each captured tank had a unique serial number. With careful observation, the Allies were able to determine the serial numbers had a pattern denoting the order of tank production. Using this data, the Allies created a mathematical model to determine the rate of German tank production. They used it to estimate that the Germans produced 255 tanks per month between the summer of 1940 and the fall of 1942.

Turns out the serial-number methodology was spot on. After the war, internal German data put der Führer’s production at 256 tanks per month—one more than the estimate.

{ Wired | Continue reading }

It is the greatest question in computer science. A negative answer would likely give a fundamentally deeper understanding of the nature of computation. And a positive answer would transform our world: Computers would acquire mind-boggling powers such as near-perfect translation, speech recognition and object identification; the hardest questions in mathematics would melt like butter under computation’s power; and current computer security methods would be as easy to crack as a TSA-approved suitcase lock.

So when Vinay Deolalikar, a computer scientist at Hewlett Packard labs in India, sent an email on August 7 to a few top researchers claiming that P doesn’t equal NP — thereby answering this question in the negative and staking a claim on the million-dollar Millennium Prize offered by the Clay Mathematics Institute — it sent shock waves through the community. Usually, computer scientists groan when they find such a claim in their Inbox, expecting the typical amateurish “proof” with the same hoary errors. But Deolalikar is a recognized and published scientist, and his paper had novel ideas from promising areas of research.

{ Science News | Continue reading }

Singapore math is taking hold in schools throughout the country. Here in New York City, home to the nation’s largest school system, a small but growing number of schools have adopted this approach, based on Singapore’s national math system.

Many teachers and parents here say Singapore math helps children develop a deeper understanding of numbers and math concepts than they gain through other math programs.

{ NY Times | Continue reading }

artwork { Jasper Johns, 0 through 9, 1960 }

The first thing to remember about probability questions is that everyone finds them mind-bending, even mathematicians. The next step is to try to answer a similar but simpler question so that we can isolate what the question is really asking.

So, consider this preliminary question: “I have two children. One of them is a boy. What is the probability I have two boys?”

This is a much easier question, though a controversial one as I later discovered. After the gathering ended, Foshee’s Tuesday boy problem became a hotly discussed topic on blogs around the world. The main bone of contention was how to properly interpret the question. The way Foshee meant it is, of all the families with one boy and exactly one other child, what proportion of those families have two boys?

To answer the question you need to first look at all the equally likely combinations of two children it is possible to have: BG, GB, BB or GG. The question states that one child is a boy. So we can eliminate the GG, leaving us with just three options: BG, GB and BB. One out of these three scenarios is BB, so the probability of the two boys is 1/3.

Now we can repeat this technique for the original question. Let’s list the equally likely possibilities of children, together with the days of the week they are born in. Let’s call a boy born on a Tuesday a BTu. Our possible situations are:

▪ When the first child is a BTu and the second is a girl born on any day of the week: there are seven different possibilities.

▪ When the first child is a girl born on any day of the week and the second is a BTu: again, there are seven different possibilities.

▪ When the first child is a BTu and the second is a boy born on any day of the week: again there are seven different possibilities.

▪ Finally, there is the situation in which the first child is a boy born on any day of the week and the second child is a BTu – and this is where it gets interesting. There are seven different possibilities here too, but one of them – when both boys are born on a Tuesday – has already been counted when we considered the first to be a BTu and the second on any day of the week. So, since we are counting equally likely possibilities, we can only find an extra six possibilities here.

Summing up the totals, there are 7 + 7 + 7 + 6 = 27 different equally likely combinations of children with specified gender and birth day, and 13 of these combinations are two boys. So the answer is 13/27, which is very different from 1/3.

It seems remarkable that the probability of having two boys changes from 1/3 to 13/27 when the birth day of one boy is stated – yet it does, and it’s quite a generous difference at that. In fact, if you repeat the question but specify a trait rarer than 1/7 (the chance of being born on a Tuesday), the closer the probability will approach 1/2.

Which is surprising, weird… and, to recreational mathematicians at least, delightfully entertaining.

{ Magic numbers: A meeting of mathemagical tricksters | NewScientist | Continue reading }

photo { Bill Owen }

I am a nerd. This fact was quite apparent to many of those around me growing up, but came as quite a surprise to me. Part of the reason that I was regarded as a nerd was because I wasn’t into sports. (…) Being a nerd, of course, I developed my love through study, through reading. (…)

Through reading, sports ceased to be a private vocabulary—one that every other boy seemed to have had whispered into his ear at infancy, but which had strangely been denied to me—and became instead a new intellectual problem, something else to be considered and solved.

The thrill of sports is and will always be largely visceral. I would have it no other way. But behind the moments of raw action are endless intricacies, seemingly limitless geometries of movement which can be studied and enjoyed in precisely the same way one enjoys science, math or history. I’m sure some people are probably reading that sentence in horror–the division between jock and nerd is so elementary and animalistic I’m surprised Joseph Campbell never wrote about it–but I mean merely that intellectual play in the consideration of sports is little different than in any other subject. There is something universal in the basic pleasure of applying mind to (subject) matter and slowly, gradually, feeling the unknown become the familiar.

{ Freddie deBoer/Wunderkammer | Continue reading }

In the realm of public policy, we live in an age of numbers. To hold teachers accountable, we examine their students’ test scores. To improve medical care, we quantify the effectiveness of different treatments. There is much to be said for such efforts, which are often backed by cutting-edge reformers. But do wehold an outsize belief in our ability to gauge complex phenomena, measure outcomes and come up with compelling numerical evidence? A well-known quotation usually attributed to Einstein is “Not everything that can be counted counts, and not everything that counts can be counted.” I’d amend it to a less eloquent, more prosaic statement: Unless we know how things are counted, we don’t know if it’s wise to count on the numbers.

The problem isn’t with statistical tests themselves but with what we do before and after we run them.

{ NY Times | Continue reading }

{ A Jefferson County teacher picked the wrong example when he used as­sassinating President Bar­ack Obama as a way to teach angles to his geome­try students. Someone alerted autho­rities and the Corner High School math teacher was questioned by the Secret Service, but was not taken into custody or charged with any crime. | Alabama Live | full story }

Many creatures demonstrate various kinds of collective behavior: birds flock, fish shoal, cattle herd and even humans collaborate from time to time.

Determining the dynamics of this kind of behavior is a hot problem that has lead to a number of fundamental discoveries in recent years. Who would have imagined that bacterial colonies cooperate when they grow, that shoals of fish can make collective decisions and that an insect swarm can act seemingly as one? And yet the mathematics that describe these systems demonstrate how easily this kind of behavior can emerge.

Today, the mathematics of animal synchrony takes a cloven-footed step forward with the unveiling of a model that describes the collective behavior of cows.

Cows are well know for their collective behavior: they tend to either all lie down or all stand up for example. Jie Sun at Clarkson University in New York state and colleagues say that this behavior can be modelled by thinking of cows as simple oscillators: they either stand or lie and do this in cycles. These oscillators are also coupled: one form of coupling may be that a cow is more likely to lie down if those around it are lying down and vice versa.

The result is a mathematical model in which the collective behavior of cows can be studied in abstract.

{ The Physics arXiv Blog | Continue reading }

I like the study in that it makes the point very clearly that, yes, there are differences between boys and girls (e.g. girls have ovaries and monthly cycles; boys do not), but, no, mathematical ability is not one of them.

{ Twenty-2-Five | Continue reading }

For better or for worse, science has long been married to mathematics. Generally it has been for the better. Especially since the days of Galileo and Newton, math has nurtured science. Rigorous mathematical methods have secured science’s fidelity to fact and conferred a timeless reliability to its findings.

During the past century, though, a mutant form of math has deflected science’s heart from the modes of calculation that had long served so faithfully. Science was seduced by statistics, the math rooted in the same principles that guarantee profits for Las Vegas casinos. Supposedly, the proper use of statistics makes relying on scientific results a safe bet. But in practice, widespread misuse of statistical methods makes science more like a crapshoot.

It’s science’s dirtiest secret: The “scientific method” of testing hypotheses by statistical analysis stands on a flimsy foundation. Statistical tests are supposed to guide scientists in judging whether an experimental result reflects some real effect or is merely a random fluke, but the standard methods mix mutually inconsistent philosophies and offer no meaningful basis for making such decisions. Even when performed correctly, statistical tests are widely misunderstood and frequently misinterpreted. As a result, countless conclusions in the scientific literature are erroneous, and tests of medical dangers or treatments are often contradictory and confusing.

{ ScienceNews | Continue reading }

Information theory is a branch of applied mathematics and electrical engineering involving the quantification of information. (…)

A key measure of information in the theory is known as entropy, which is usually expressed by the average number of bits needed for storage or communication. Intuitively, entropy quantifies the uncertainty involved when encountering a random variable. For example, a fair coin flip (2 equally likely outcomes) will have less entropy than a roll of a die (6 equally likely outcomes).

{ Wikipedia | Continue reading }

Claude Elwood Shannon (1916 – 2001), an American electronic engineer and mathematician, is known as “the father of information theory.”

Shannon is famous for having founded information theory with one landmark paper published in 1948. But he is also credited with founding both digital computer and digital circuit design theory in 1937, when, as a 21-year-old master’s student at MIT, he wrote a thesis demonstrating that electrical application of Boolean algebra could construct and resolve any logical, numerical relationship. It has been claimed that this was the most important master’s thesis of all time. (…)

The Las Vegas connection: Information theory and its applications to game theory
Shannon and his wife Betty also used to go on weekends to Las Vegas with M.I.T. mathematician Ed Thorp, and made very successful forays in blackjack using game theory type methods co-developed with physicist John L. Kelly Jr. based on principles of information theory. They made a fortune, as detailed in the book Fortune’s Formula. (…)

Shannon and Thorp also applied the same theory, later known as the Kelly criterion, to the stock market with even better results.

{ Wikipedia | Continue reading | Mathematical Theory of Claude Shannon, A study of the style and context of his work up to the genesis of information theory. | PDF }

recto/verso { Welcome to Fabulous Las Vegas sign, 1959, designed by Betty Willis. | In hopes that the design would be used freely, Willis never copyrighted her sign’s design. | PBS | Continue reading | More Betty Willis | NY Times | Photos: The Neon Museum, Las Vegas }

The mathematics that describe both sensory perception and the transmission of information turn out to have remarkable similarities.

In 1834, the German physiologist Ernst Weber carried out a series of experiments to determine the limits of sensory perception. He gave a blindfolded man a mass to hold and gradually increased its weight, asking the subject to indicate when he first became aware of the change.

These experiments showed that the smallest increase in weight that a human can perceive is proportional to the initial weight. The German psychologist Gustav Fechner later interpreted Weber’s work as a way of measuring the relationship between the physical magnitude of a stimulus and its perceived intensity.

The resultant mathematical model of this process is called the Weber-Fechner law and shows that the relationship between the stimulus and perception is logarithmic. The Weber-Fechner law is important because it established a new field of study called psychophysics. (…)

The logarithmic relationship between a stimulus and its perception crops up in various well known examples such as the logarithmic decibel scale for measuring sound intensity and a similar logarithmic scale for measuring the visible brightness of stars, their magnitude.

Today, Hi Jun Choe, a mathematician at Yonsei University in South Korea, says there is an interesting connection between the Weber-Fechner Law and the famous mathematical theory of information developed by Claude Shannon at Bell Labs in the 1940s.

Shannon’s work is among the most important of the 20th century. It establishes the limits on the amount of information that can be sent from one location in the universe to another. It is no exaggeration to say that the world’s entire computing and communications infrastructure is based on Shannon’s work. (…)

Of course, the idea that sensory perception is a form of communication and so obeys the same rules, is not entirely surprising. What’s astonishing (if true) is that the connection has never been noticed before.

{ The Physics arXiv Blog | Continue reading }

{ Using a mathematical concept called sparsity, the compressed-sensing algorithm takes lo-res files and transforms them into sharp images. | Wired | Full story }

A new mathematical model of hurricane formation finally solves one of the outstanding puzzles of climate change but also predicts dramatic increases in the number of storms as the world warms.

{ The Physics arXiv Blog | Continue reading }

photo { Christophe Kutner }

The Galton board, also known as a quincunx or bean machine, is a device for statistical experiments named after English scientist Sir Francis Galton. It consists of an upright board with evenly spaced nails (or pegs) driven into its upper half, where the nails are arranged in staggered order, and a lower half divided into a number of evenly-spaced rectangular slots. The front of the device is covered with a glass cover to allow viewing of both nails and slots. In the middle of the upper edge, there is a funnel into which balls can be poured, where the diameter of the balls must be much smaller than the distance between the nails. The funnel is located precisely above the central nail of the second row so that each ball, if perfectly centered, would fall vertically and directly onto the uppermost point of this nail’s surface.

Each time a ball hits one of the nails, it can bounce right (or left) with some probability P (and q = 1 - P). For symmetrically placed nails, balls will bounce left or right with equal probability, so P = q = 1/2. If the rows are numbered from 0 to ^{N - 1}, the path of each falling ball is a Bernoulli trial consisting of ^N steps. Each ball crosses the bottom row hitting the ⁿth peg from the left (where ^{0≤ n ≤ N - 1}) If and only if it has taken exactly ⁿ right turns, which occurs with probability

{ Wolfram MathWorld | Continue reading }

from the archives { The whole system—the nine thousand polystyrene balls dropping through a pegboard of 330 precisely cantilevered nylon pins, the real-time photoelectric counters tallying (by LED readout) the segmented heaps forming below, the perennially balky bucket-conveyor for resetting an experimental run—had all been painstakingly constructed and calibrated in order first to exemplify, and then to defy, what the Victorian statistician Francis Galton dubbed the “Law of Frequency of Error.” | Cabinet | Continue reading }

We use coin tosses to settle disputes and decide outcomes because we believe they are unbiased with 50-50 odds.

Yet recent research into coin flips has discovered that the laws of mechanics determine the outcome of coin tosses: The startling finding is they aren’t random. Instead, for natural flips, the chance of a coin coming up on the same side as it started is about 51 percent. Heads facing up predicts heads; tails facing up predicts tails.

{ The Big Money | Continue reading }

{ The 13 Archimedean solids are the convex polyhedra that have a similar arrangement of nonintersecting regular convex polygons of two or more different types arranged in the same way about each vertex with all sides the same length. | Wolfram MathWorld | Continue reading }

In November 2002, an obscure Russian mathematician named Grigori Perelman caused a sensation in the mathematical community when he posted the first in a series of papers proving the most famous unsolved problem in topology: the Poincaré conjecture. He caused another sensation four years later when he was awarded the Fields medal - the “mathematics Nobel” - for his work, declined to accept it, and then left mathematics altogether. When last heard of, he was living a reclusive existence at his mother’s home in St Petersburg.

{ NewScientist | Continue reading }

Russian math prodigy Grigory Perelman should be a celebrated millionaire. Instead, he is a poor recluse who lives with his mother.

In 2006, Sir John Ball, the president of the International Mathematical Union, travelled to St. Petersburg hoping to convince Grigory Perelman to accept his place as the most celebrated mathematician alive.

Ball spent two days there, locked in an increasingly desperate argument with Perelman, a haughty, dishevelled 39-year-old. Ball asked Perelman to accept a Fields Medal, the highest award for achievement in mathematics. The Fields is given out every four years, to as few as two recipients. Perelman, the man who had solved the insoluble Poincaré Conjecture, refused the award. Four years earlier, he had turned down a $1 million prize for the same solution.

Ball first tried to convince Perelman to travel to Spain for the ceremony. Since Perelman rarely left the dilapidated flat he shared with his mother, that went nowhere. Ball suggested Perelman skip the ceremony, but accept the award. He declined again. Eventually, Ball left, baffled and frustrated. The prize was awarded to Perelman anyway.

{ The Star | Continue reading | Perelman in a Subway [pics] }

related { It may be no accident that, while some of the best American mathematical minds worked to solve one of the century’s hardest problems—the Poincaré Conjecture—it was a Russian mathematician working in Russia who, early in this decade, finally triumphed. | Wall Street Journal }

Taxicab geometry, considered by Hermann Minkowski in the 19th century, is a form of geometry in which the usual metric of Euclidean geometry is replaced by a new metric in which the distance between two points is the sum of the (absolute) differences of their coordinates.

The taxicab metric is also known as Manhattan distance, or Manhattan length, with corresponding variations in the name of the geometry. The latter names allude to the grid layout of most streets on the island of Manhattan, which causes the shortest path a car could take between two points in the city to have length equal to the points’ distance in taxicab geometry.

{ Wikipedia | Continue reading }

{ The hairy ball theorem of algebraic topology states that there is no nonvanishing continuous tangent vector field on the sphere. The theorem was first stated by Henri Poincaré in the late 19th century, and first proved in 1912 by Brouwer. | Wikipedia | Continue reading }

{ Luitzen Egbertus Jan Brouwer (1881-1966) was a Dutch mathematician and philosopher, usually cited as L. E. J. Brouwer but known to his friends as Bertus | Wikipedia | Continue reading }